Lower Bound for Spectral Estimation in Noisy Super-Resolution

Abstract and 1 Introduction

1.1 ESPRIT algorithm and central limit error scaling

1.2 Contribution

1.3 Related work

1.4 Technical overview and 1.5 Organization

2 Proof of the central limit error scaling

3 Proof of the optimal error scaling

4 Second-order eigenvector perturbation theory

5 Strong eigenvector comparison

5.1 Construction of the “good” P

5.2 Taylor expansion with respect to the error terms

5.3 Error cancellation in the Taylor expansion

5.4 Proof of Theorem 5.1

A Preliminaries

B Vandermonde matrice

C Deferred proofs for Section 2

D Deferred proofs for Section 4

E Deferred proofs for Section 5

F Lower bound for spectral estimation

References

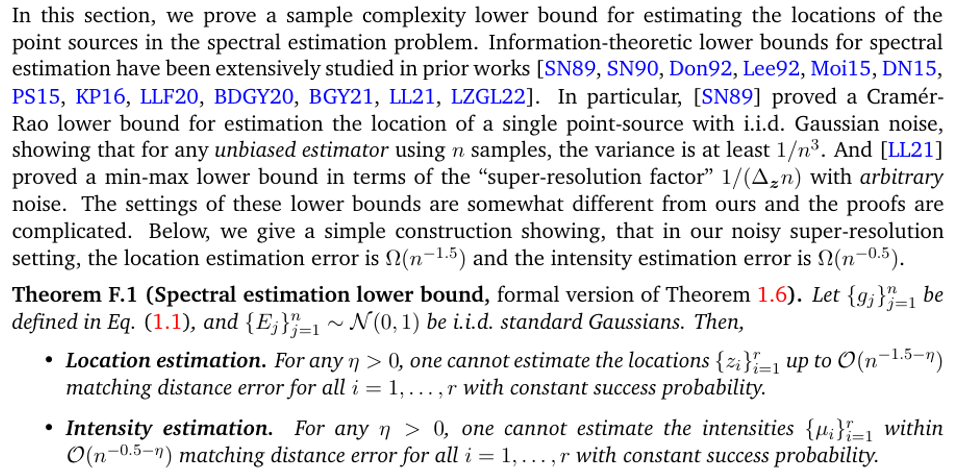
\ To prove this theorem, we will employ the following lemma [AAL23, Thm. 1.8]:

\

\

:::info This paper is available on arxiv under CC BY 4.0 DEED license.

:::

:::info Authors:

(1) Zhiyan Ding, Department of Mathematics, University of California, Berkeley;

(2) Ethan N. Epperly, Department of Computing and Mathematical Sciences, California Institute of Technology, Pasadena, CA, USA;

(3) Lin Lin, Department of Mathematics, University of California, Berkeley, Applied Mathematics and Computational Research Division, Lawrence Berkeley National Laboratory, and Challenge Institute for Quantum Computation, University of California, Berkeley;

(4) Ruizhe Zhang, Simons Institute for the Theory of Computing.

:::

\